Torricelli’s trumpet is not counterintuitive
Description
There is nothing counterintuitive about an infinite shape with finite volume, contrary to the common propaganda version of the calculus trope known as Torricelli’s trumpet. Nor was this result seen as counterintuitive at the time of its discovery in the 17th century, contrary to many commonplace historical narratives.
Transcript
Torricelli’s trumpet is not counterintuitive. Your calculus textbook lied to you.
You’ve probably heard of this cliché, Torricelli’s trumpet: an allegedly “paradoxical” shape that has infinite area but finite volume. It’s staple example in calculus textbooks. Well, there’s nothing to it, in my opinion. It’s a propaganda lie. Let’s revisionist-history the heck out this thing.
<figure class="wp-block-image size-large">
 </figure>
</figure>I will tell you why there’s nothing counterintuitive about this result. Then I’ll argue that it was not seen as counterintuitive at the time, in the 17th century, contrary to what everyone tells you. Then I will explain, in terms of the sociology of the mathematical community, why this myth is still so popular. That is to say, why it is such a comforting myth to so many people, despite being wrong.
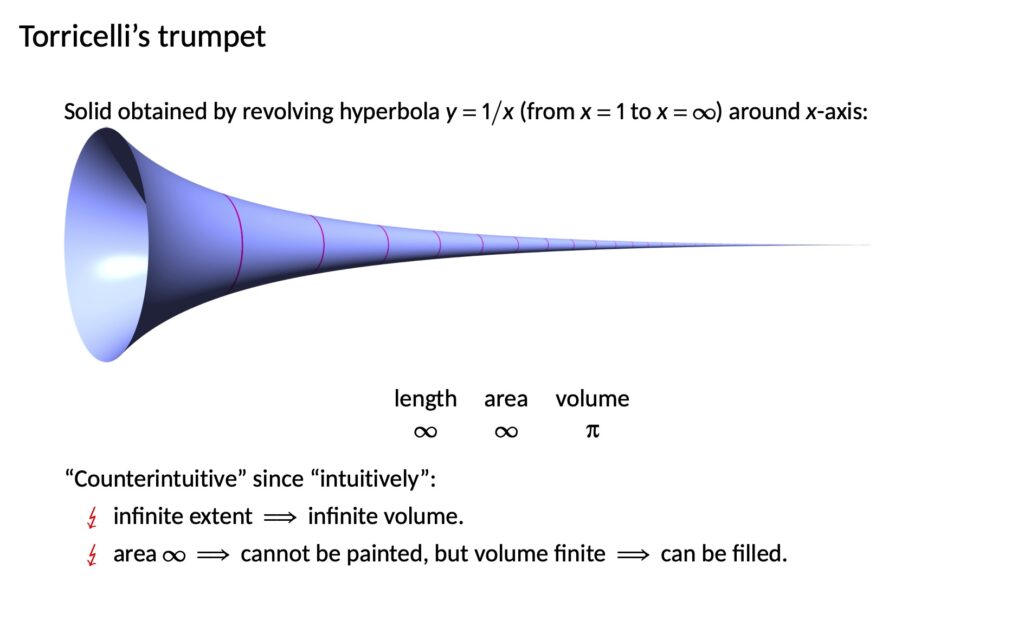
So, the trumpet. You take the hyperbola y=1/x and you rotate it about the x-axis. It makes a trumpet shape, a kind of funnel that becomes infinitely narrow the further you go.
Also known as “Gabriel’s Horn.” Actually I tried to look up the origin of this silly name but I couldn’t find it. I guess it was perhaps coined for the American market? “Torricelli” is bit too “Euro” isn’t it? Now, “Gabriel’s Horn” on the other hand, there you have a nice pious biblical name.
Anyway, whatever you want to call it. The volume of the funnel is finite. For example from x=1 onwards. Despite its area and its length being infinite.
There are two supposed contradictions here. On the one hand, finite volume with infinite extent (infinite length) could be regarded as two contradictory properties that it would be surprising to find in the same solid, allegedly. Alternatively, finite volume with infinite area could also be seen as a clash of two incompatible properties: a shape having those two properties at the same time is supposed to be contrary to “intuition,” allegedly. Sometimes it is put like this: Such a solid cannot be painted, since it has infinite area, yet it can be filled with paint, since it has finite volume. But I think this can be a misleading move that mixes the issue of Torricelli’s trumpet with general issues of how infinite processes correspond to everyday experience, which is perhaps a separate source of so-called “counterintuitive” phenomena. The fact is that if Torricelli’s trumpet is supposed to be a surprising result, then the source of the contradiction is supposed to be the properties of volume, area, and length of this shape, and not some secondary intuitions about infinite processes in general.
In my opinion, the properties of Torricelli’s trumpet are not “counterintuitive.”
<figure class="wp-block-image size-large">
 </figure>
</figure>First, is it counterintuitive for a finite amount of paint to cover an infinite surface? Of course not. Let’s put it like this. Suppose you have a can of paint. Obviously it contains a finite volume of paint, such as one liter. Now, open the can and pour the paint on the floor. Think of a big floor, like a basketball court. Get a spatula and start spreading the paint across as much area as you can.
Where do you think this process will stop? This is mathematical paint. You can spread it as thin as you like. How much area can you cover, if you can spread the paint thinner and thinner and thinner?
What does your “intuition” tell you? Does your “intuition” say that this spreading process with terminate after a certain number of square meters of the floor painted? Of course not. That would be idiotic.
And yet that is precisely what the standard account of Torricelli’s trumpet would have you believe. It is supposed to be “counterintuitive”, the story goes, for a finite volume of paint to cover an infinite area. Well, we have just seen that that premise is idiotic. Obviously a finite amount of paint can spread further and further, as long as you make it thinner and thinner. There is nothing “counterintuitive” about that.
Why would “intuition” say that the process of spreading the paint thinner and thinner would suddenly terminate at some finite bound? Why on earth would it? What would be the “intuitive” reason for why you could spread paint thinner, and then thinner, and then thinner, and then thinner, and then not thinner all of a sudden? Where would this invisible upper bound come from? Why would “intuition” stipulate the existence of such a ghost? It makes no sense.
<figure class="wp-block-image size-large">
 </figure>
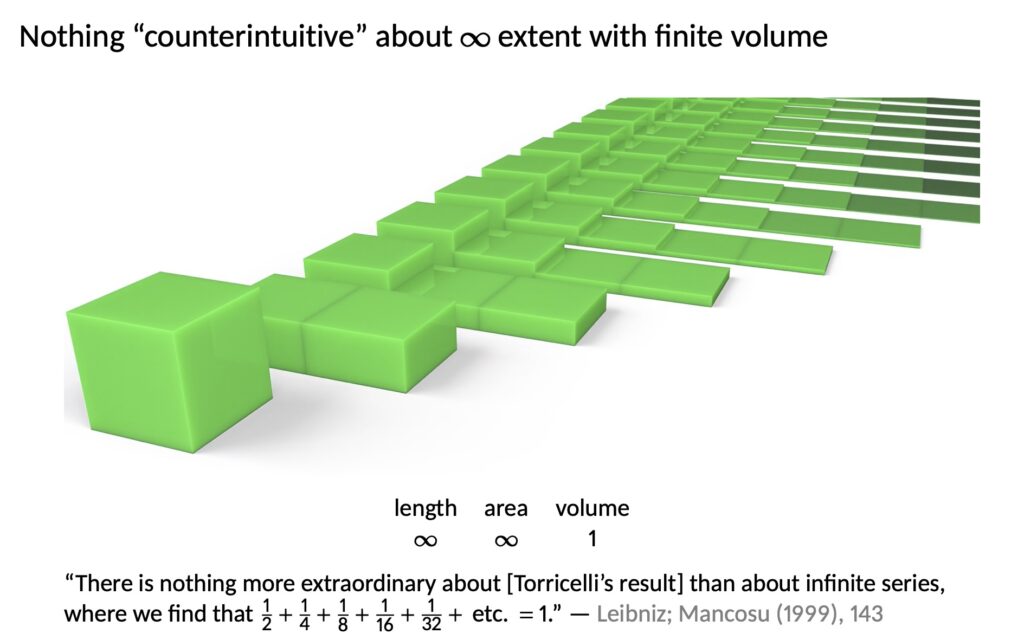
</figure>Here’s another thought experiment that proves the same thing. We’re not using paint anymore, but rather a cube. You have a cube of unit volume in front of you. You cut it in half, horizontally. Like a sandwich. Then you place the top half side-by-side with the bottom half. Next cut the top half in half again in the same manner, and bring the new top slice down next to the bottom slice. So now you have a kind of stairway with three steps.
Continue in the same way: you keep bisecting the last piece and placing all the pieces in a row. All the slices have a length and a width of 1, the original dimensions of the cube, because you are always cutting horizontally. You are cutting the heights in half, leaving the width and the breadth of all the pieces the same throughout.
So you get a row of blocks that each have a length and width of 1, and whose heights are 1/2, 1/4, 1/8, 1/16, and so on. The total volume remains 1 throughout the process of course, because you only moved the existing volume around without adding to it or taking anything away. Meanwhile, both the length and the area of the combined shape clearly approaches infinity, as is intuitively clear.
So with this simple intuitive argument we get infinite extent with finite volume. So clearly infinite extent with finite volume is by no means contrary to intuition.
Note that this simple thought experiment also shows that Torricelli’s trumpet is not a case of a technical mathematical result being philosophically or qualitatively different from simple common-sense examples, contrary to what calculus teachers like to pretend. So the standard story is wrong in two ways. It claims that advanced calculus proves intuition wrong. But that’s doubly a lie. First, intuition is not wrong, and furthermore you don’t need fancy calculus to show any of this anyway. The simple thought experiments of the repeatedly bisected cube or the painted basketball court, which you can explain to a 5-year-old, contain everything that is relevant. There is nothing qualitatively new added by the use of calculus.
These things were also well understood historically. For example, Isaac “Barrow … clearly saw that [Torricelli’s] theorem can be intuitively explained by the fact that ‘the infinite diminution of one dimension compensates the infinite increase of the other’.” This fits with the basketball court example. Although Barrow was talking about the relationship between volume and length, not volume and area. But the point is the same.
The equivalent of the bisected cube example was also well understood. For example, Leibniz rightly remarked that “there is nothing more extraordinary about [Torricelli’s result] than about infinite series, where we find that 1/2 + 1/4 + 1/8 + 1/16 + 1/32 etc. = 1.” Exactly. Nothing to see here. Just as Leibniz says.
Let’s turn to





